I can use the exponent rules to simplify expressions 292753

For rules of exponents applied to algebraic functions instead of numerical examples, read Rules of Exponents Algebraic The laws of exponents are rules that can be applied to combine and simplify expressions with exponents These rules are true if a a a is positive, and m m m and n n n are real numbersThe Power Rule for Exponents Let's simplify (52)4 In this case, the base is 52 and the exponent is 4, so you multiply 52 four times (52)4 = 52 • 52 • 52 • 52 = 58 (using the Product Rule – add the exponents) (52)4 is a power of a power It is the fourth power of 5 to the second powerFree Exponents Calculator Simplify exponential expressions using algebraic rules stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy Learn more Accept Solutions

Quotient Rule For Exponents To Simplify Fractions Krista King Math Online Math Tutor

I can use the exponent rules to simplify expressions

I can use the exponent rules to simplify expressions-X x or y y y is not equal to zero, we can definitely apply the zero rule of exponent here as well This gives us ( 2 x 2 y) 0 = 1 {\left ( {2 {x^2}y} \right)^0} = 1 (2x2y)0 = 1 Simplify the exponential expression below Each expression with a parenthesis raised to the power of zero, 0Correct answer Explanation In order to solve this problem, each of the answer choices needs to be simplified Instead of simplifying completely, make all terms into a form such that they have 100 as the exponent Then they can be easily compared Double exponent use braces to clarify

Exponent Rules Helping With Math

Learning Objectives Product and Quotient Rules Use the product rule to multiply exponential expressions Use the quotient rule to divide Use the product rule to multiply exponential expressions Use the quotient rule to divide exponential expressions The Power Rule for Exponents Use the power rule👉 Learn how to simplify expressions using the power rule and the negative exponent rule of exponents When several terms of an expression is raised to an exTo simplify complicated radical expressions, we can use some definitions and rules from simplifying exponents Recall the Product Raised to a Power Rule from when you studied exponents This rule states that the product of two or more nonzero numbers raised to a power is equal to the product of each number raised to the same power

Simplifying Expressions Using Exponents Displaying top 8 worksheets found for Simplifying Expressions Using Exponents Some of the worksheets for this concept are More properties of exponents, Properties of exponents, Exponent rules practice, Simplifying expressions with negative exponents math 100, Exponent and radical rules day , Algebraic expressions packet, ExponentAlgebra 1 Day 1 and 2 Lesson Plan Recalling the Laws of Exponents Standards 8EEI1 Understand and apply the laws of exponents (ie, product rule, quotient rule, power to a power, product to a power, quotient to a power, zero power property, negative exponents) to simplify numerical expressions that include integer exponentsAccording to exponent rules, when we divide the expressions we _______ the exponents Q According to exponent rules, when we raise the power to a power we _______ the exponents Q According to exponent rules, when we multiply the expressions we _______ the exponents Q Simplify the exponential expression

2 Use the product rule for e xponents 3 Use the power rule for e xponents 4 Use the power rules for products and Quotients 5 Use the Quotient rule for exponents, and Define a Number raised to the 0 power 6 Decide Which rule(s) to Use to Simplify an expression 51 Exponents Evaluating Exponential ExpressionsExpanding the expression using the definition produces multiple factors of the base, which is quite cumbersome, particularly when n is large For this reason, we will develop some useful rules to help us simplify expressions with exponents In this example, notice that we could obtain the same result by adding the exponentsExponent Rules Through the rules of exponents, you can calculate values with high powers Many of the arithmetic operations like addition, subtraction, multiplication, and division can be conveniently be performed in quick steps using the exponential rules For using these exponential rules across two exponents, the base of the exponents should be equal

How To Use Exponent Rules Exponent Rules Simplifying Expressions Exponents

Simplifying With Rational Exponents Section 6 1 2 Ppt Download

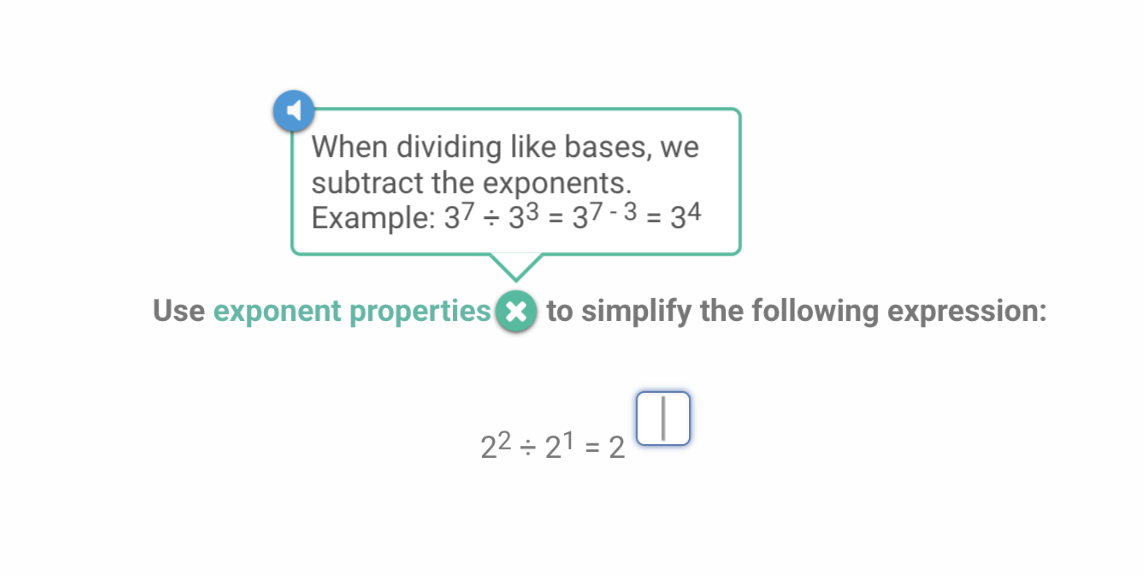
Apr 17, 10 · The video shows how to solved mixed property problems using the exponent rules See if there are any rules you can apply to the problem until you get to a point where you can't simplify anymore The instructor shows in the example problem that the two bases are the same, but there is extras happening the numerator The power to power multiply rule is appliedSimplify exponential expressions with positive and/or negative exponents Multiply or divide expressions in scientific notation Evaluate polynomials for specific values apply all the rules of exponents to simplify expressions Exponent Rules ProductDec 14,  · Multiply two numbers with exponents by adding the exponents together xm × xn = xm n Divide two numbers with exponents by subtracting one exponent from the other xm ÷ xn = xm − n When an exponent is raised to a power, multiply the exponents together (

Using Rules For Exponents To Simplify Expressions Mediaspace Wcsu

Solved Problems 0 1 Exponents Use The Exponent Rules To S Chegg Com

Here are some exponential properties that you should be familiar with a^ (bc) = (a^b)^c // or vice versa a^b * a^c = a^ (b c) a^b / a^c = a^ (b c) a^c * b^c = (a*b)^c Once you know those exponential properties, we can use them to simplify our exponential expressions to a more simpler form, preferably A*B^tSquare root simplification calculator algebra numbers Derive a Java program that Solve a quadratic equation of the type Ask the user to enter the values of a, b and c and then print out the values of x) Note You can calculate the values by using the quadratic formula and using methods of Math class from Java APISolution (a) 21 2 ⋅ 25 2 The bases are the same, so we add the exponents 21 25 2 Add the fractions 26 2 Simplify the exponent 23 Simplify 8 2 1 2 · 2 5 2 The bases are the same, so we add the exponents 2 1 2 5 2 Add the fractions 2 6 2 Simplify the exponent 2 3 Simplify 8 (b)

Negative And Zero Exponents

What Are The Laws Of Exponents Video

Let's get some practice simplifying hairy expressions that have exponents in them and so we have a hairy expression right over here and I encourage you to pause the video and see if you can rewrite this in a in a simpler way all right let's work through this together and the first thing that jumps out at me is the numerator here I have number 125 raised to the 1/8 power times the same👉 Learn how to simplify expressions using the quotient rule of exponents The quotient rule of exponents states that the quotient of powers with a common baJan ,  · It means using the definition of exponents, as Purple Math states, by rewriting our exponential expression so that we can clearly see the number or variable being multiplied by itself several times This will lay the framework for our Exponent Rules when we rewrite expressions using a base with a single exponent

Rules Of Exponents

Solved Example 1 Simplify The Given Expression Using The Chegg Com

Simplify Rational Expressions worksheet We can use one of the laws of exponents to explain how fractional exponents work As you probably already know $$ \sqrt{9} \cdot \sqrt{9} = 9 $$ Well, let's look at how that would work with rational (read fraction ) exponents Since we now know $$ \sqrt{9} = 9^{\frac 1 2 } $$Test your ability to simplify expressions using exponents in this quiz/worksheet combo You will have five practice problems to solve, and you will need to know how to use the product of powersOct 04, 19 · Move it to the denominator, remove the negative, and simplify from there If you have a negative exponent in the denominator, move it

Rules For Exponents Beginning Algebra

Rules For Exponents Beginning Algebra

Solutions with detailed explanations to Questions on Simplifying Exponents are presented The rules of of exponents are applied to solve the following questions 27(1 9)2(92 35) = Solution Use the quotient rule of exponents (a b)m = am bm to rewrite (1 9)2 in the given expression as 27(1 9)2(92 35) = 27 12 92 92 35 Rewrite 27 as 33This makes it easier to identify the like bases before using the Product Property of Exponents Example Simplify (m4n − 3)(m − 5n − 2) ( m 4 n − 3) ( m − 5 n − 2) Solution (m4n − 3)(m − 5n − 2) ( m 4 n − 3) ( m − 5 n − 2) Use the Commutative Property to get like bases together m4m − 5 ·Laws Of Exponents In Mathematics, there are different laws of exponents All the rules of exponents are used to solve many mathematical problems which involve repeated multiplication processes The laws of exponents simplify the multiplication and division operations and help to solve the problems easily

Algebra Simplify An Expression With Exponents Youtube

Exponents 30 Task Cards Simplifying Expressions Set 2 Difficult

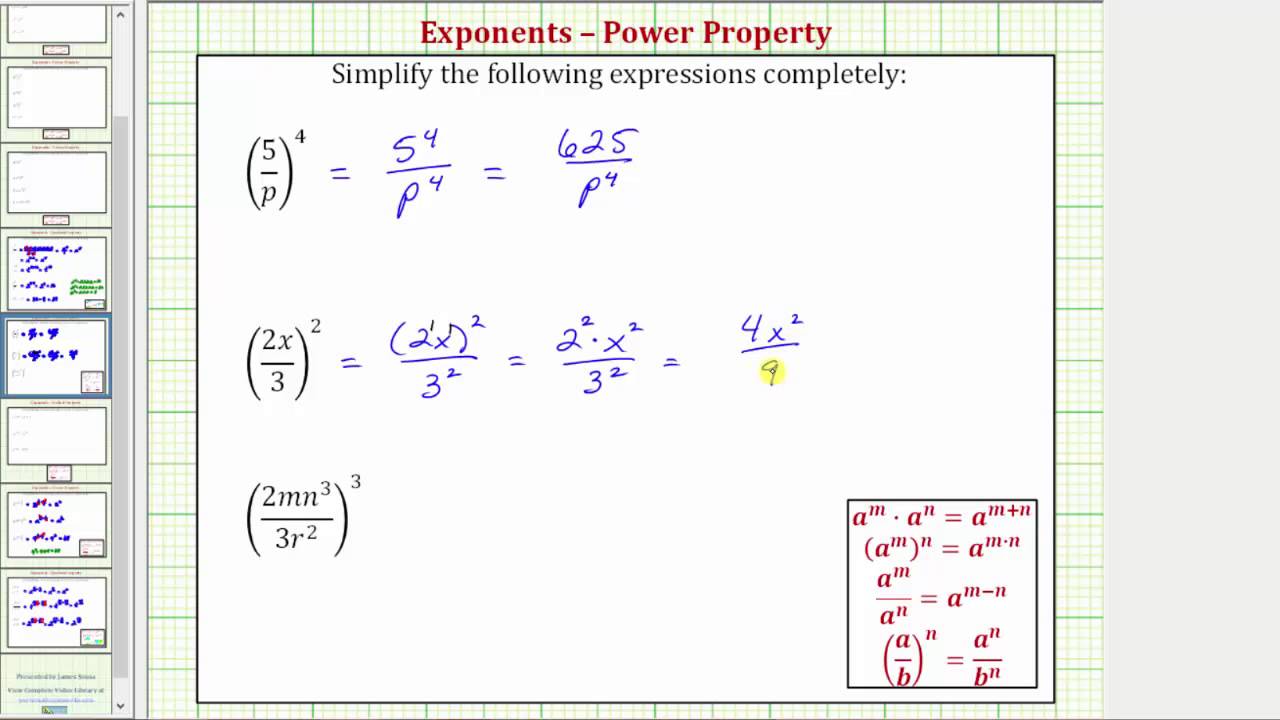
Simplifying expressions using the Laws of Exponents We can use what we know about exponents rules in order to simplify expressions with exponents When simplifying expressions with exponents we use the rules for multiplying and dividing exponents, and negative and zero exponents Simplifying expressions with exponentsUse the power property of exponents to simplify expressions Use the product to a power property of exponents to simplify expressions You have seen that when you combine like terms by adding and subtracting, you need to have the same base with the same exponentAnswer to 1 Simplify the following using exponent rules (You can use a calculator) a (2x4) 4 (5x9) 2 b (4x5) 3 (6x4) 2 5x8y6 3 C 10x11yz

Algebra Using The Rules Of Exponents To Simplify Youtube

Exponent Rules 7 Key Strategies To Solve Tough Equations Prodigy Education

Use exponent rules to simplify the following expressions as much as possible Use only positive exponents in your final answer 2 (a) (x²y³)³ · (yx) ab (b) a³bc2Below is List of Rules for Exponents and an example or two of using each rule ZeroExponent Rule a 0 = 1, this says that anything raised to the zero power is 1 Power Rule (Powers to Powers) (a m ) n = a mn , this says that to raise a power to a power you need to multiply the exponentsSep ,  · Use the rules of exponents to simplify the expressions Match the expression with its equivalent Brainlycom Use the rules of exponents to simplify the expressions Match the expression with its equivalent value helpagain is waiting for your help Add your answer and earn points

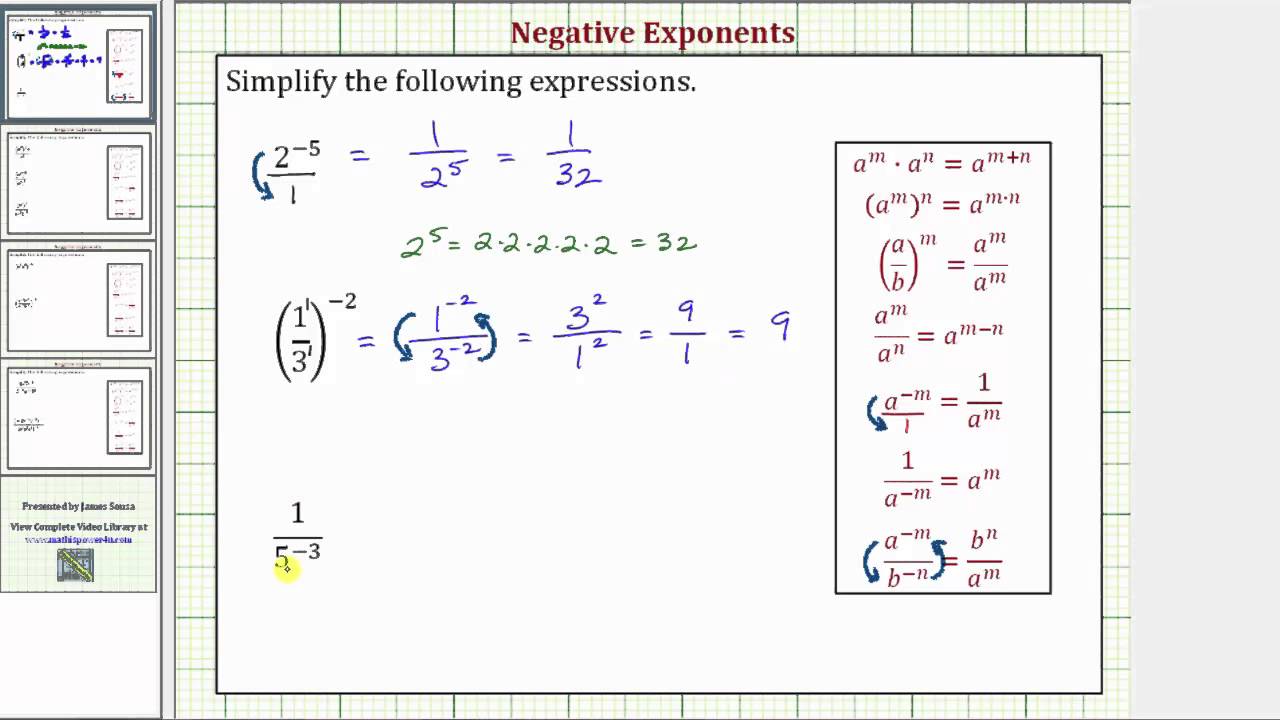
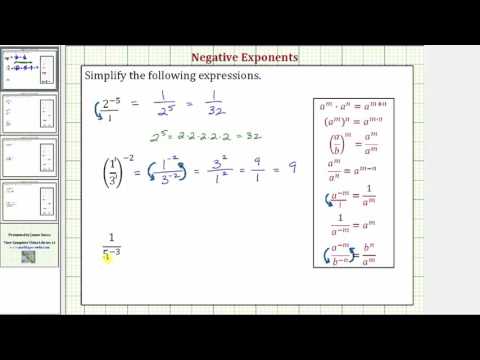
Negative Exponents

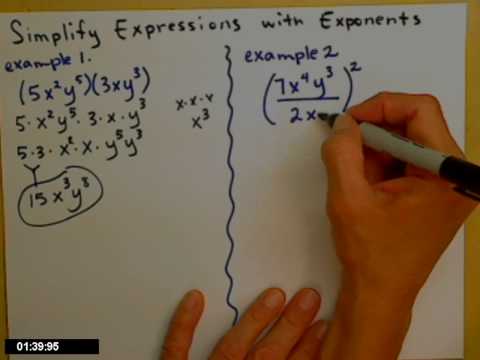
Simplifying Expressions With Exponents Youtube

Whenever you have an exponent expression that is raised to a power, you can simplify by multiplying the outer power on the inner power (xm) n= xm n If you have a product inside parentheses, and a power on the parentheses, then the power goes on each element insideThis demonstrates our first exponent rule, the Product Rule when multiplying two expressions that have the same base, we can simplify the product by adding the exponents xm ⋅ xn = xm n x m ⋅ x n = x m n (521) permalink Checkpoint 523 permalink Recall that x = x1We can use what we know about exponents rules in order to simplify expressions with exponents When simplifying expressions with exponents we use the rules for multiplying and dividing exponents, and negative and zero exponents In Algebra and higher math courses such as Calculus, we will often encounter simplifying expressions with exponents

Rules For Exponents Beginning Algebra

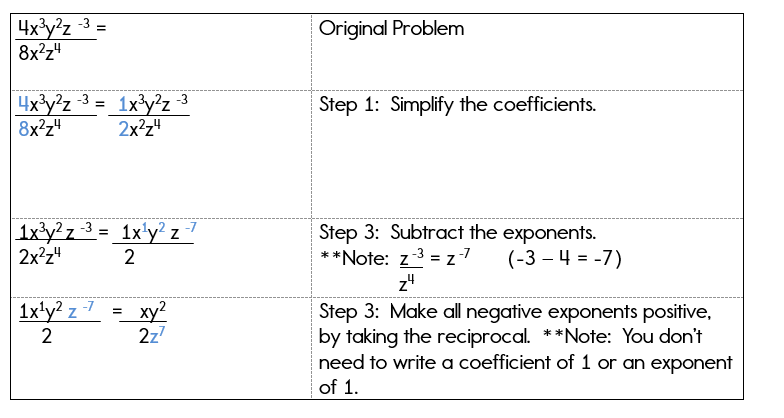
Negative And Zero Exponents

Mar 08,  · The power rule tells us that when we raise an exponential expression to a power, we can just multiply the exponents In ( 3 2) 3 (3^2)^3 ( 3 2 ) 3 , the first exponent is 2 2 2 and the second exponent is 3 3 3 The power rule tells us that we can just multiply those exponents and get 2 ⋅ 3 = 6 2\cdot3=6 2 ⋅ 3 = 6, which means thatOct 22, 16 · Simplify the following expressions a) b) c) Solution Since there are just numbers and no variables, we can evaluate the expressions and get rid of the exponents completely a) We can use the quotient rule first and then evaluate the result OR we can evaluate each part separately and then divideWe already know how to add, subtract, and multiply But, just as Dua Lipa has some New Rules, we have new ones of our own that we need to learn in order to simplify exponent expressions product rules, quotient rules, and power rules Try out these rules in our product rule, quotient rule, and power rule calculators ⬇️

4 3 Rules For Exponents Mathematics Libretexts

Solved Simplify The Exponential Expression D30 List The E Chegg Com

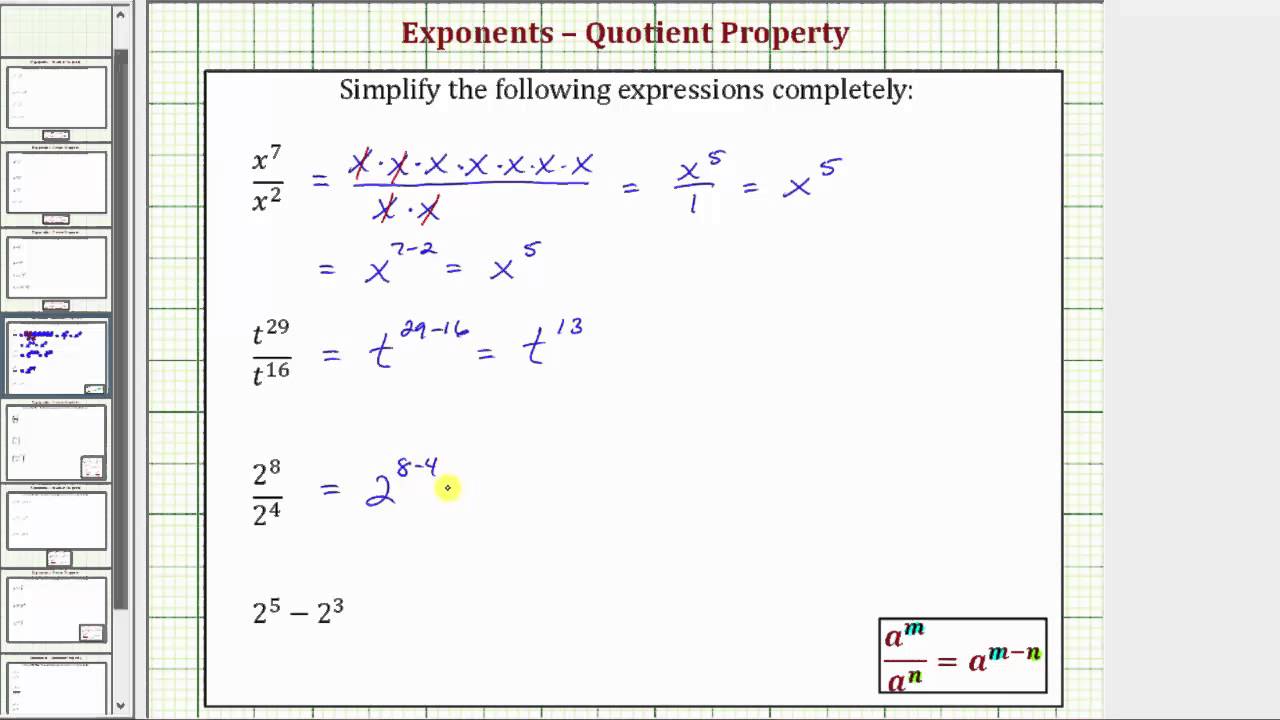
Rules For Exponents Beginning Algebra

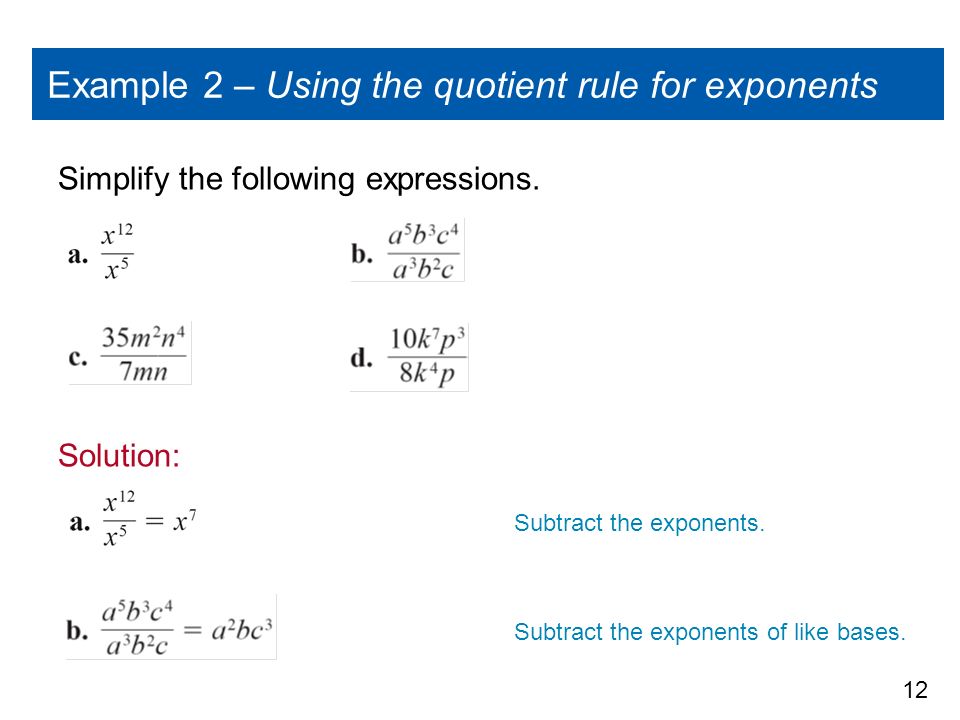
Copyright C Cengage Learning All Rights Reserved 3 Exponents Polynomials And Functions Ppt Download

Exponent Rules Helping With Math

Use Power Rules To Simplify Expressions With Negative Exponents Module 6 Youtube

Negative Exponents Exponent Rules

Simplify Expressions Using The Power Rule Of Exponents Basic Youtube

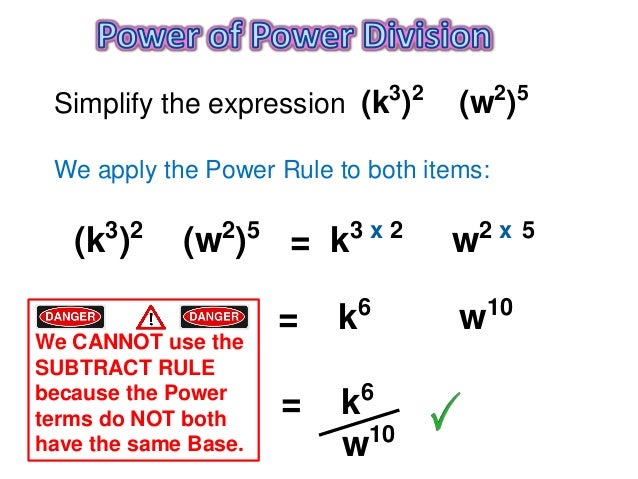
Power Of Power Exponent Rule

Exponent Rules Christmas Practice Simplifying Evaluating Expressions

Exponent Rules Simplifying Expressions Color By Number Exponent Rules Simplifying Expressions Middle School Math Resources

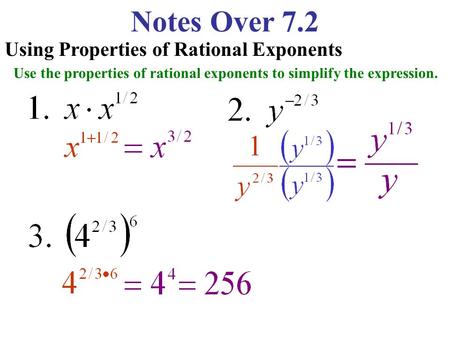
Properties Of Rational Exponents And Radicals Ppt Video Online Download

How To Do Negative Exponents 25 Amazing Examples Negative Exponents Exponents Math Methods

Exponents Simplifying Expressions Using Exponent Rules Set 1 Tpt

Pin On Teaching

Zero Exponent Rule Definition Examples Video Lesson Transcript Study Com

Exponent Rules Simplifying Expressions Color By Number Tpt

Exponent Rules Simplify Each Algebraic Expression Do Not Leave Negative Exponents Ppt Download

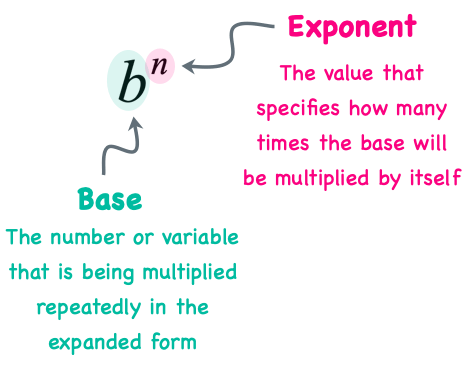
Rules Of Exponents Chilimath

Exponents Simplifying Expressions Using Exponent Rules Set 1 Tpt

4 3 Rules For Exponents Mathematics Libretexts

Applying The Laws Of Exponents Verbal Symbolic Texas Gateway

Simplifying Expressions With Exponents On Vimeo

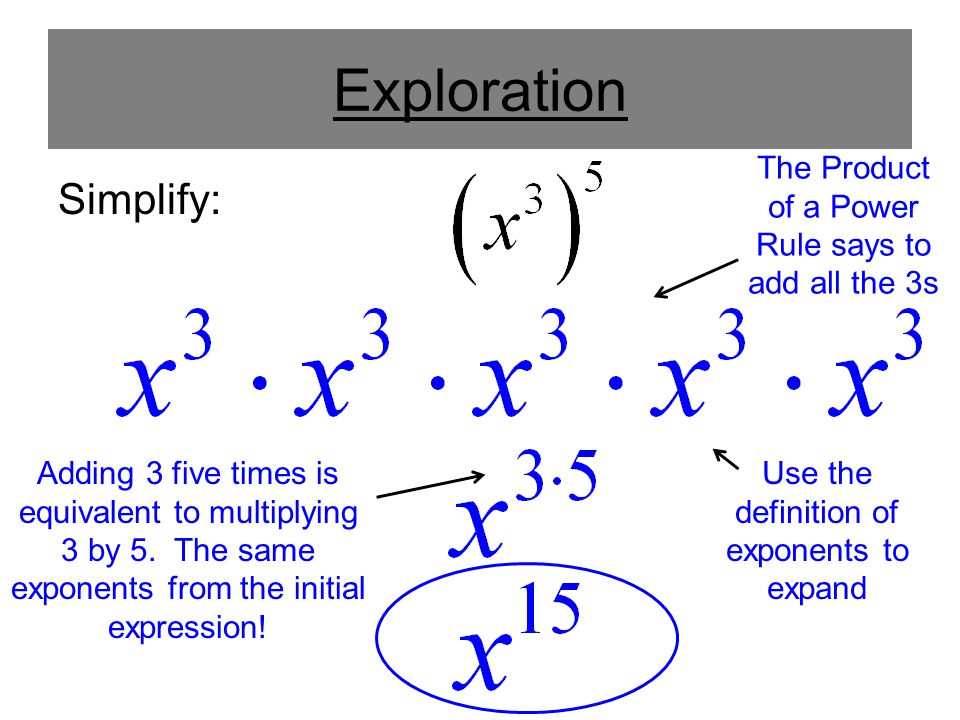
Simplifying Exponential Expressions Exponential Notation Base Exponent Base Raised To An Exponent Example What Is The Base And Exponent Of The Following Ppt Download

How To Simplify Expressions With Exponents Video Lesson Transcript Study Com

Simplifying Using Exponent Rules Youtube

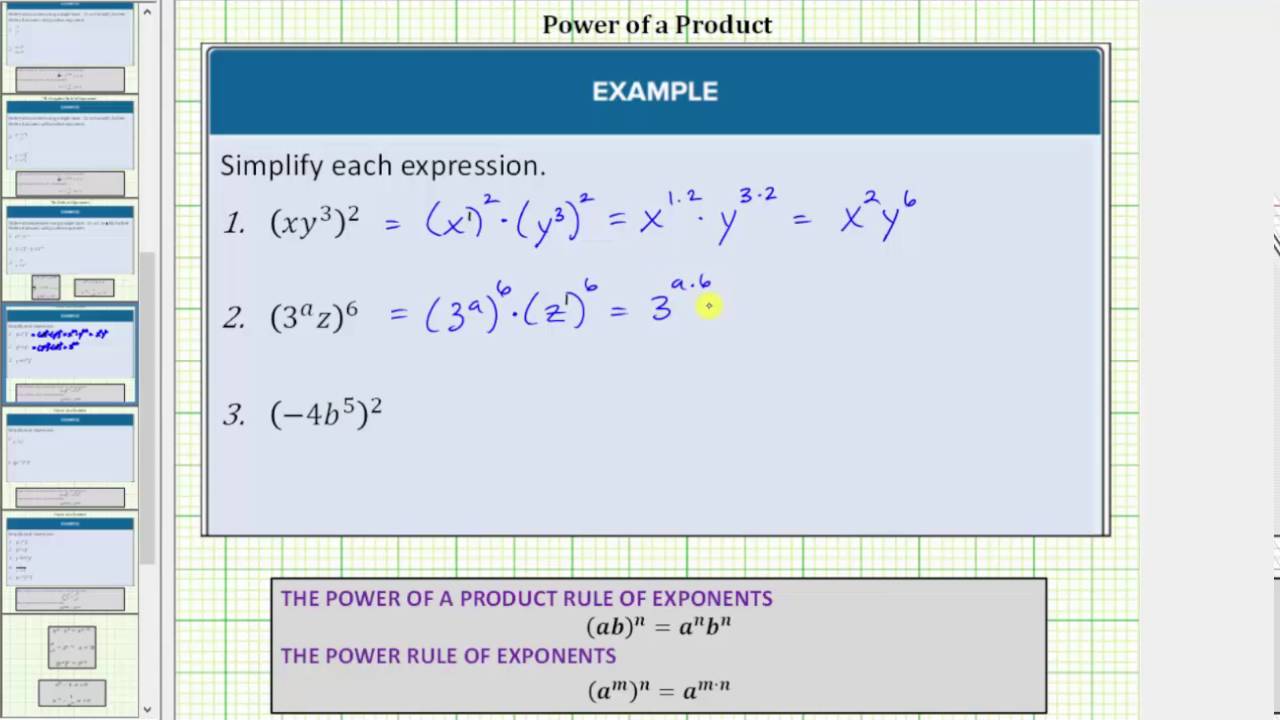
Simplify Expressions Using Exponent Rules Power Of A Product Youtube

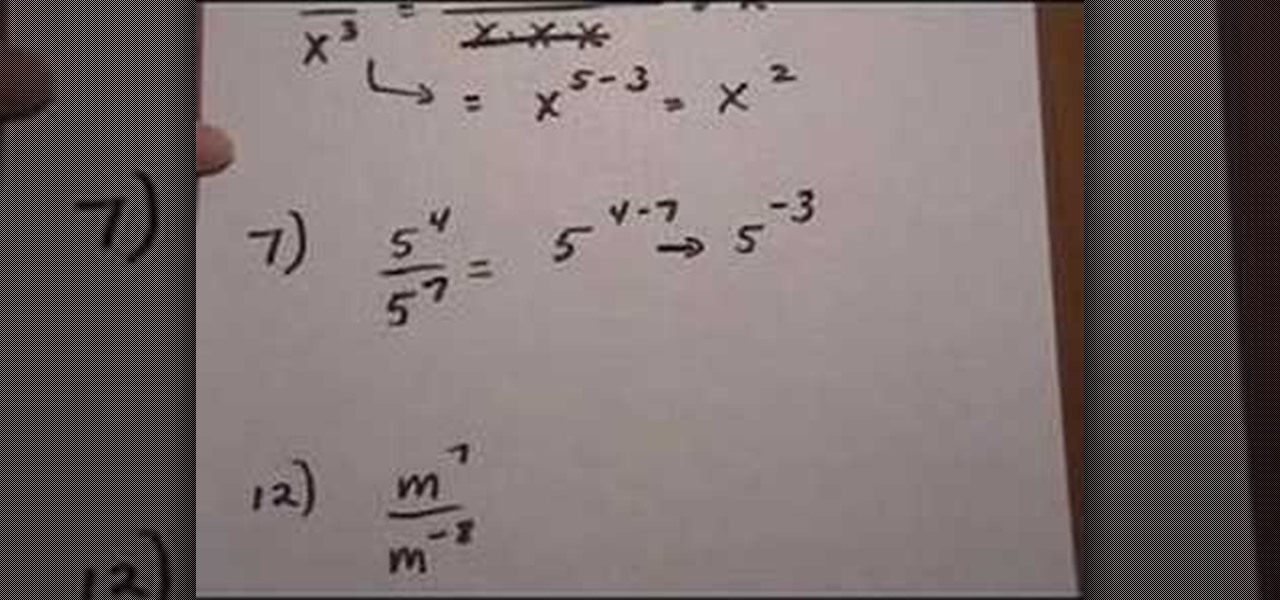
How To Simplify Exponential Expressions Via The Quotient Rule Math Wonderhowto

Exponent Rules Maze Exponent Rules Free Math Lessons Algebra Activities High School

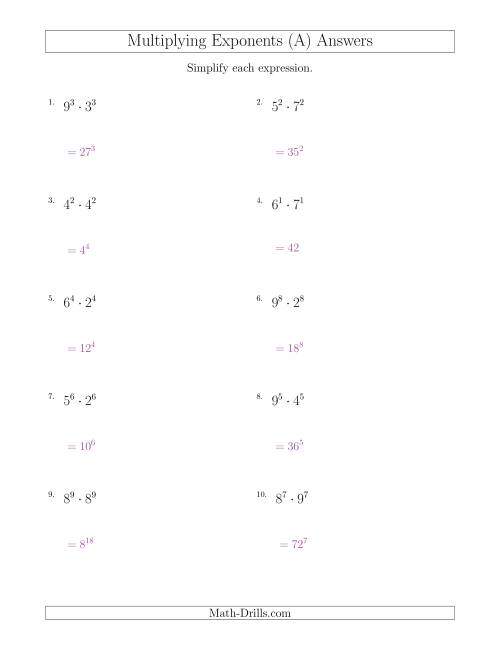
Multiplying Exponents With Different Bases And The Same Exponent All Positive A

Simplifying Exponents Circuit Math Activity Math Activities Simplifying Expressions Exponents

Ex 13 2 1 Using Laws Of Exponents Simplify And Write In Exponent

Lesson Simplifying Expressions Rules Of Exponents Nagwa

How To Simplify Expressions With Exponents Video Lesson Transcript Study Com

Simplify Expressions Using Exponent Rules Lesson Plans

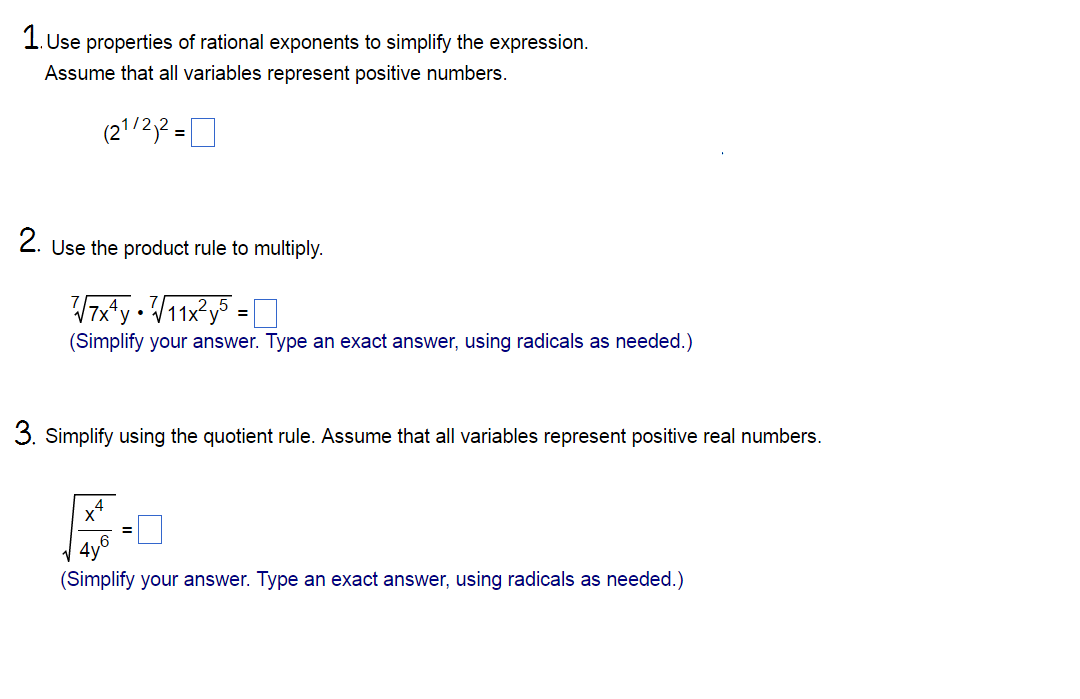
Solved Use Properties Of Rational Exponents To Simplify T Chegg Com

Part Ii Activitiesactivity 1 Directions Simplify The Following Expressions Then Identify Which Laws Brainly Ph

Use The Product Rule To Simplify The Expression By Tutorcircle Team Issuu

I Can Use The Exponent Rules To Simplify Exponential Expressions Ppt Video Online Download

How To Simplify Math Expressions 13 Steps With Pictures

Simplifying Algebraic Expressions By Brett Berry Math Hacks Medium

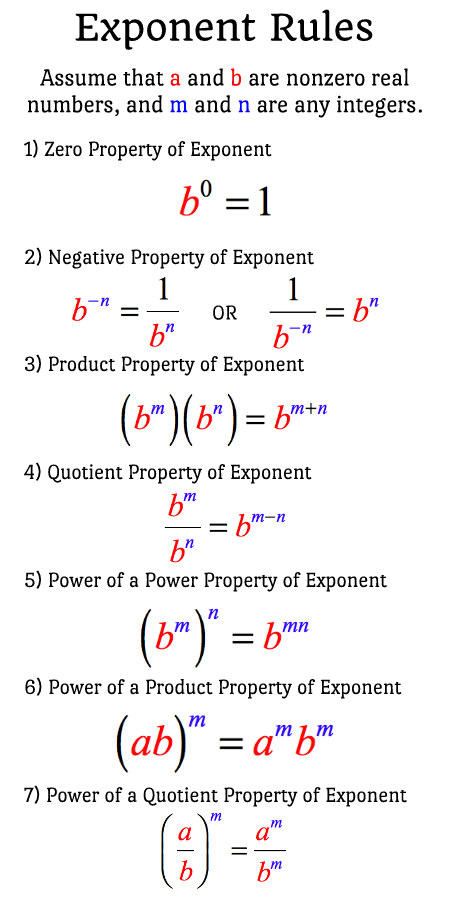
Rules Of Exponents Chilimath

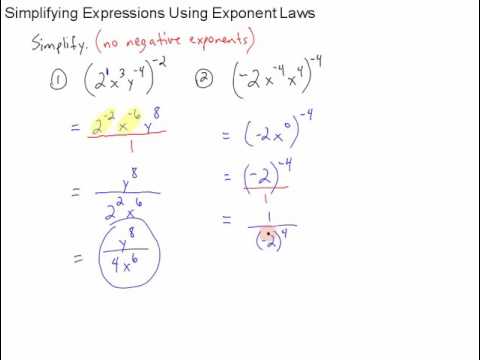
Simplifying Expressions Using Exponent Laws Youtube

Laws Of Exponents Triples Activity Exponents Exponent Rules Negative Exponent Rule

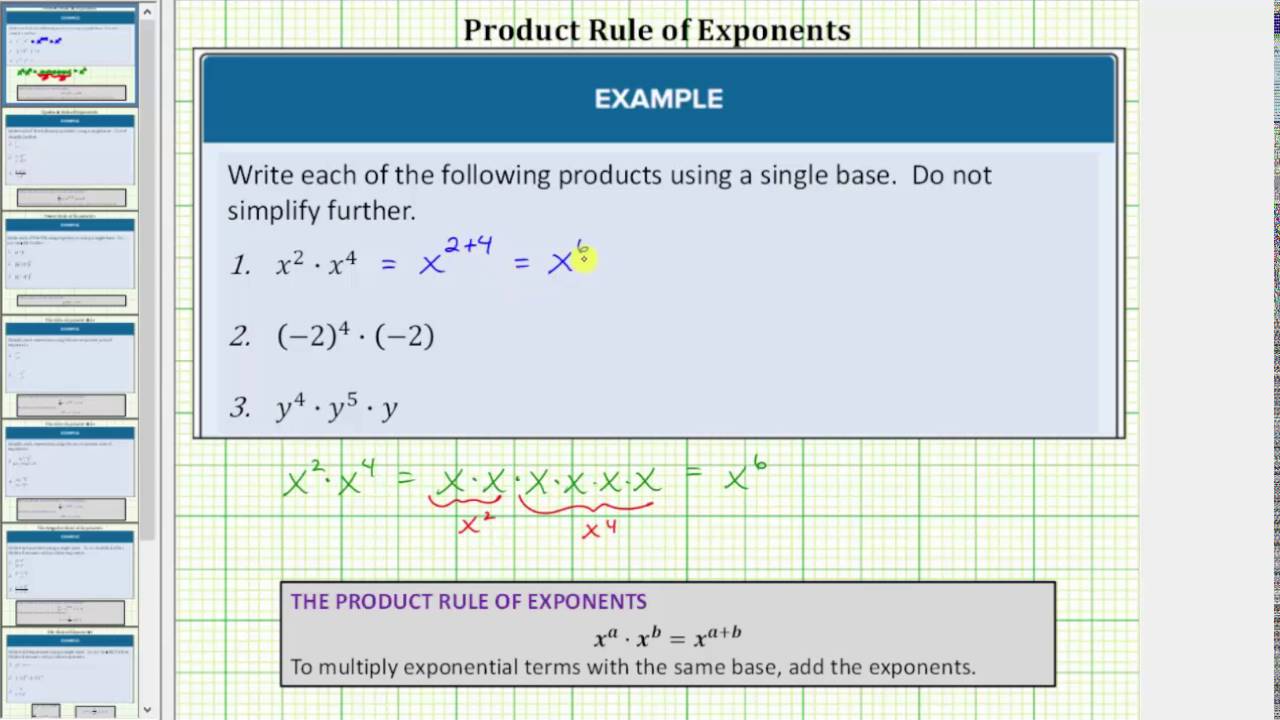
Rules Of Exponents Video Lessons Examples And Solutions

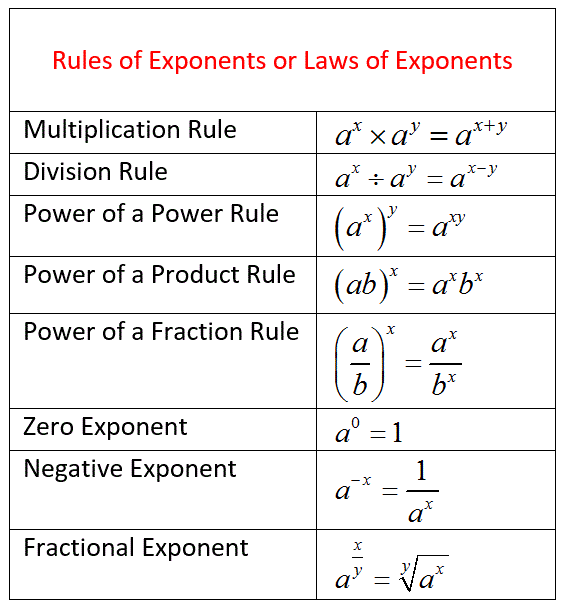
Rules Of Exponents Video Lessons Examples And Solutions

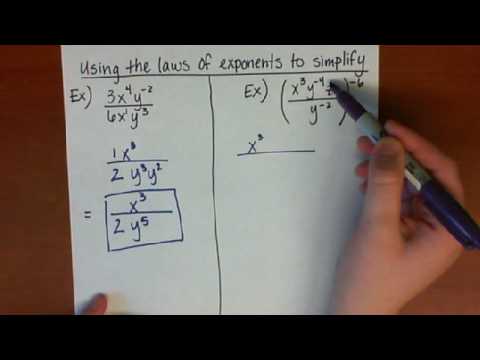
Simplifying Expressions Using The Laws Of Exponents Youtube

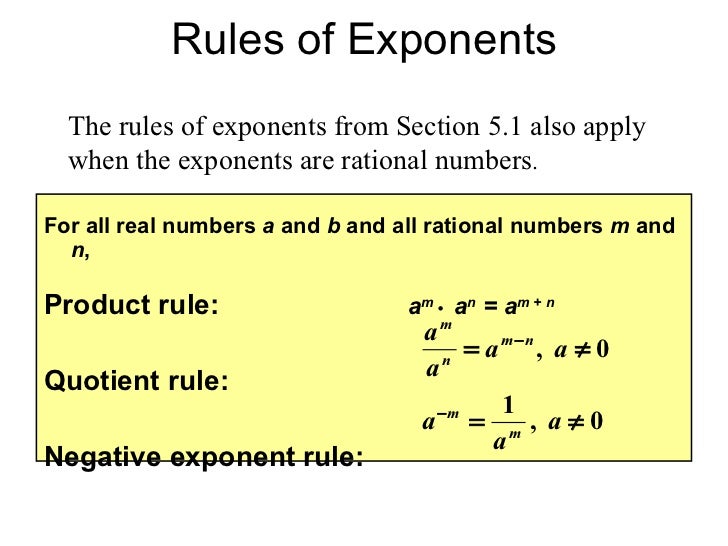
Simplifying Radical Expressions Rational Exponents Radical Equations

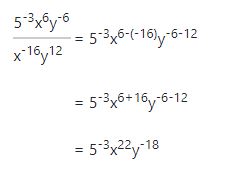
Exponent Rules Helping With Math

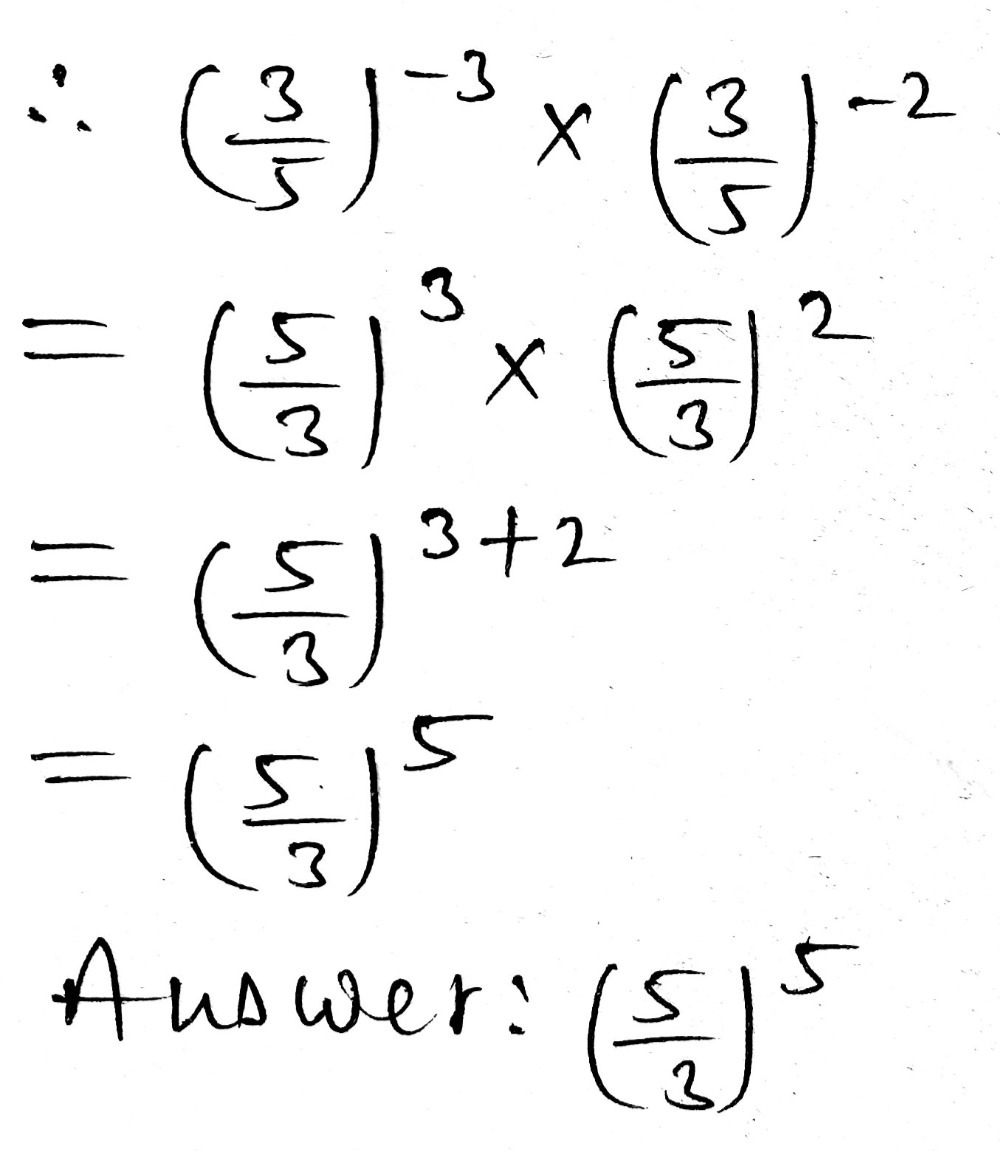
Use Exponent Rules To Simplify The Expression Exp Gauthmath

Negative Exponents

Solved 8 Simplify Each Expression Using Exponent Rules Chegg Com

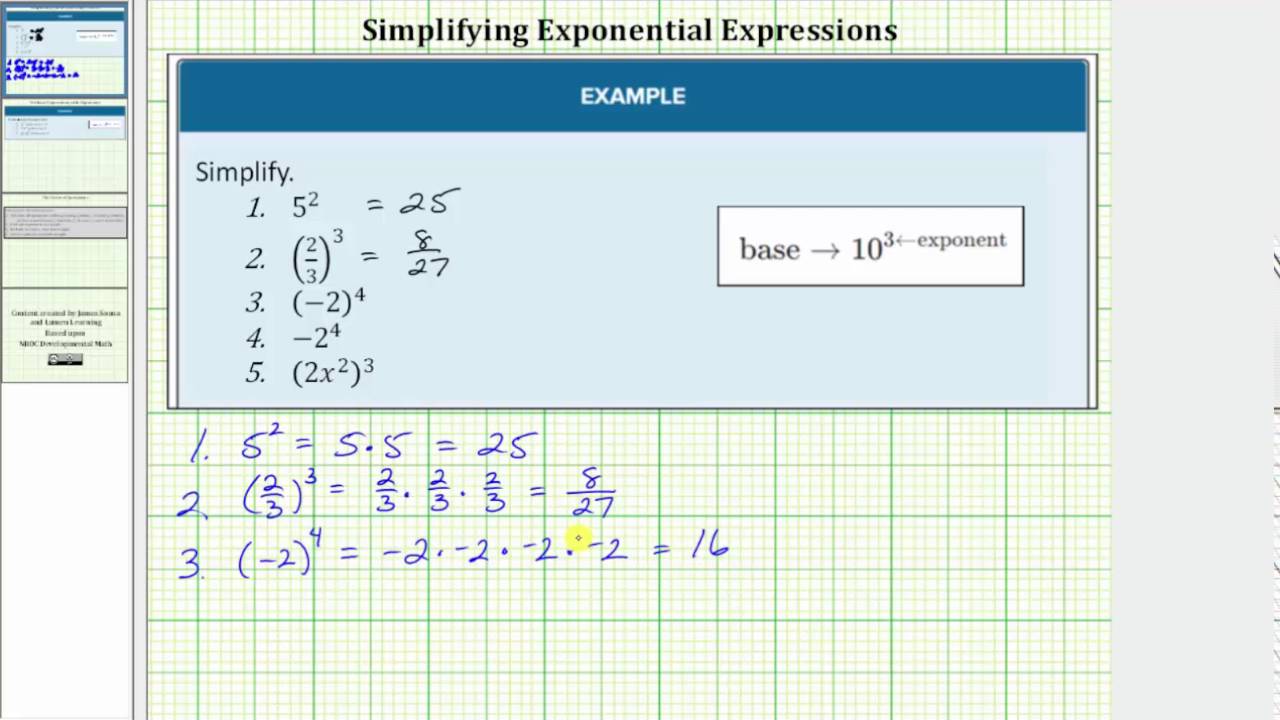
Rules For Exponents Beginning Algebra

Rules Of Exponents

Simplifying Expressions With Rational Exponents Video Lesson Transcript Study Com

Using Exponent Rules To Simplify Exponential Expressions Youtube

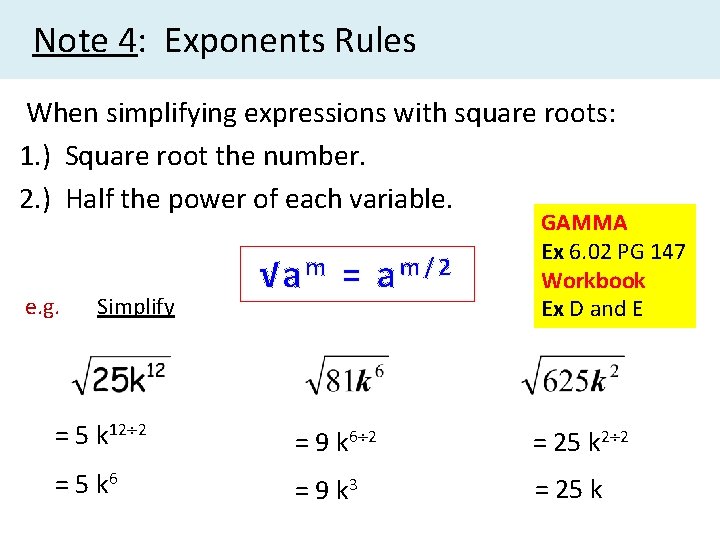
1 2 Algebra C A T External 4

Rational Exponents

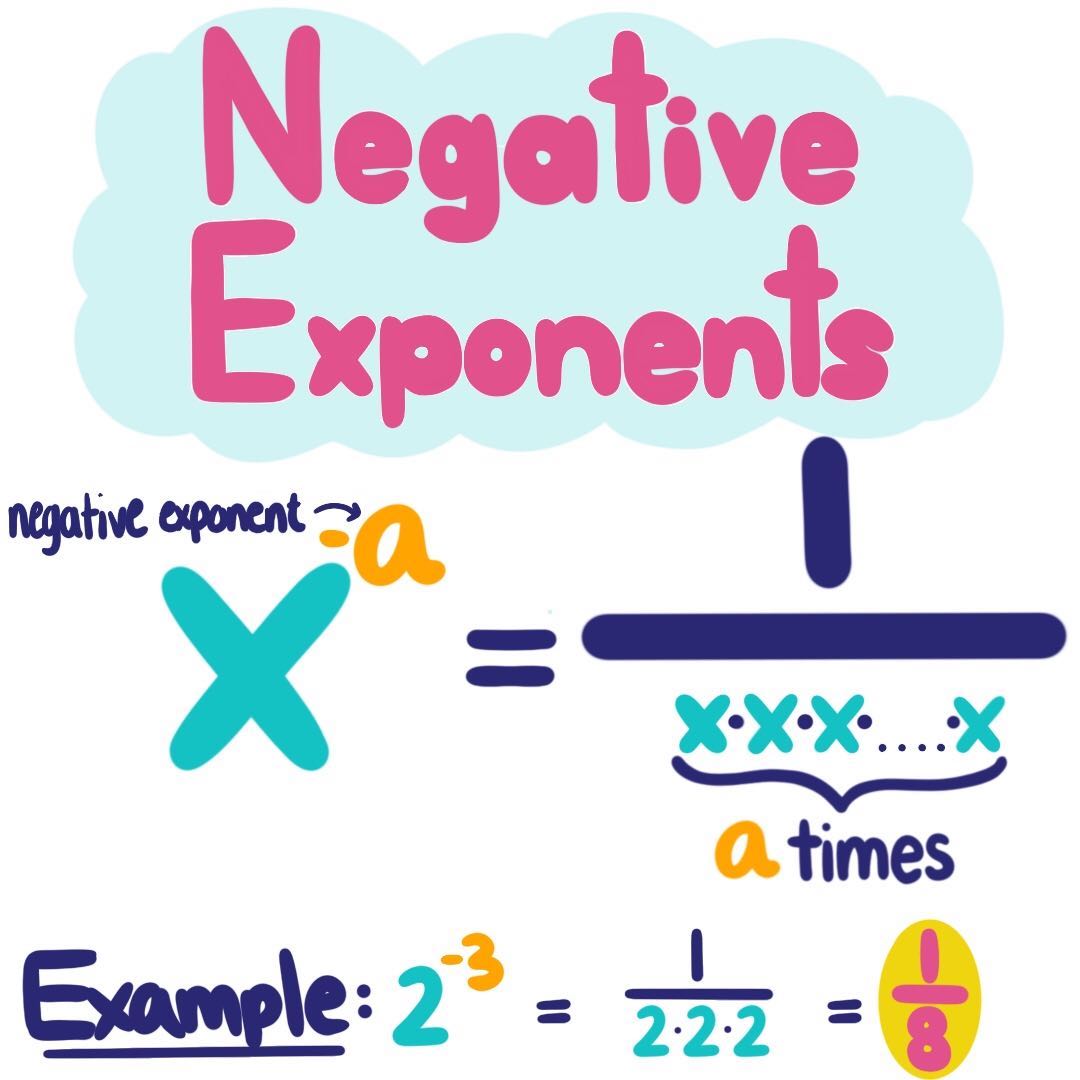
Negative Exponents Rules Examples Expii

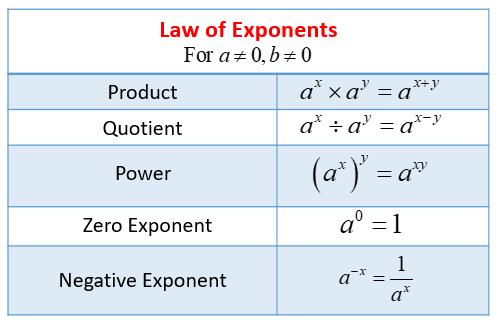
Simplifying Expressions With Exponents Examples Solutions Videos Worksheets Activities

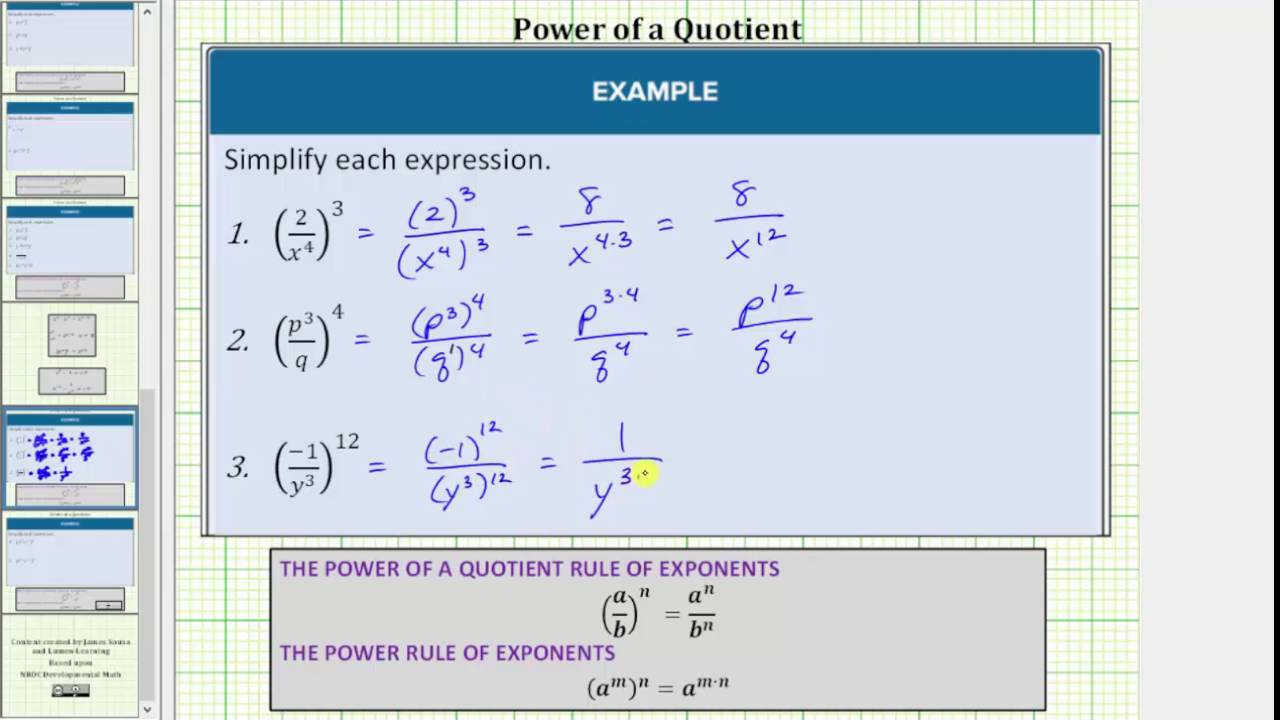
Simplify Expressions Using Exponent Rules Power Of A Quotient Youtube

Exponent Rules Helping With Math

Quotient Rule For Exponents To Simplify Fractions Krista King Math Online Math Tutor

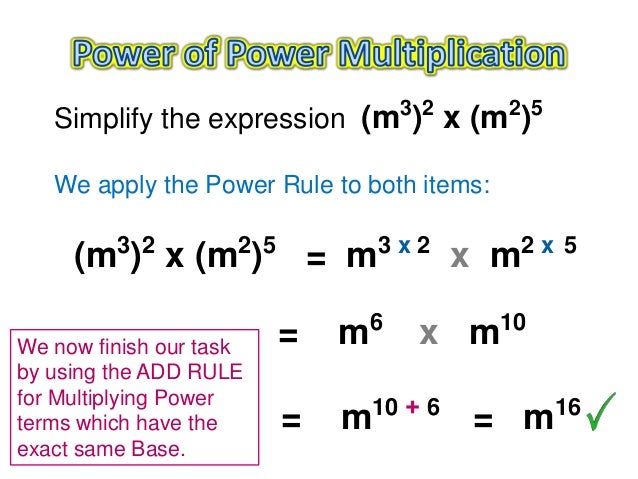
Power Of Power Exponent Rule

Rewriting Exponential Expressions As A Bᵗ Video Khan Academy

Simplifying Exponent Expressions Purplemath

How To Simplify Expressions With Exponents Video Lesson Transcript Study Com

Solved 4 1 6 Simplify Exponential Expressions Using The R Chegg Com

Using Exponent Laws To Simplify Algebraic Expressions Youtube

Exponent Rules Simplifying Expressions Color By Number Tpt

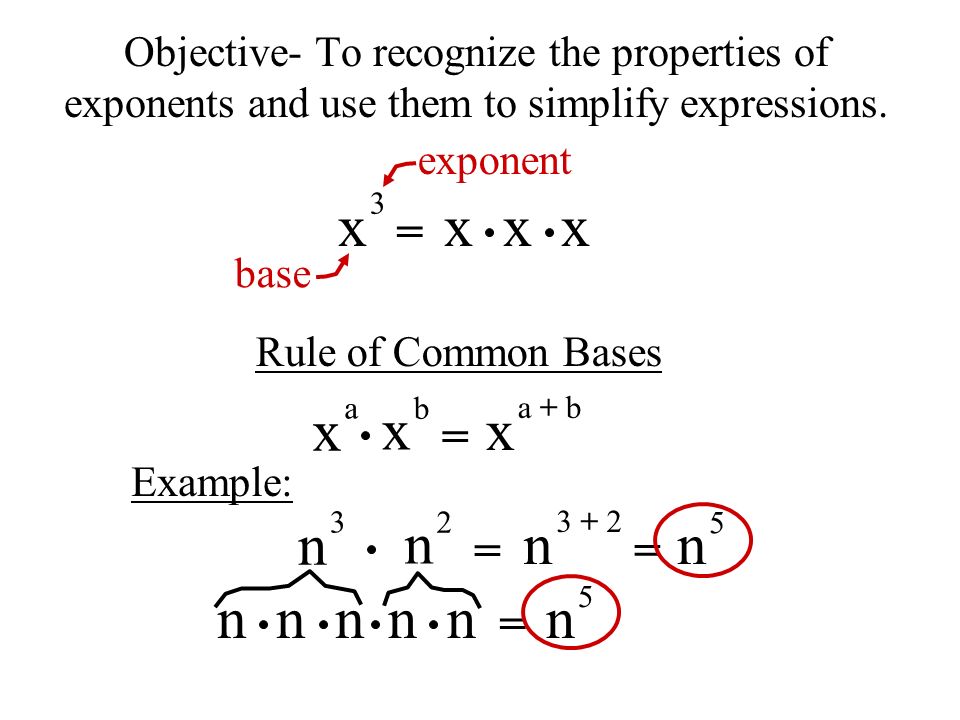
N N N N Objective To Recognize The Properties Of Exponents And Use Them To Simplify Expressions X 3 X X X Exponent Base Rule Of Common Bases X A Ppt Download

How To Solve Algebraic Problems With Exponents 8 Steps

Use The Rules Of Exponents To Simplify The Expressions Match The Expression With Its Equivalent Brainly Com

How To Simplify Expressions With Exponents Video Lesson Transcript Study Com

Simplify Expressions With Exponents Using All Exponent Rules Lessons Blendspace

Exponent Rules Simplifying Expressions Color By Number Tpt

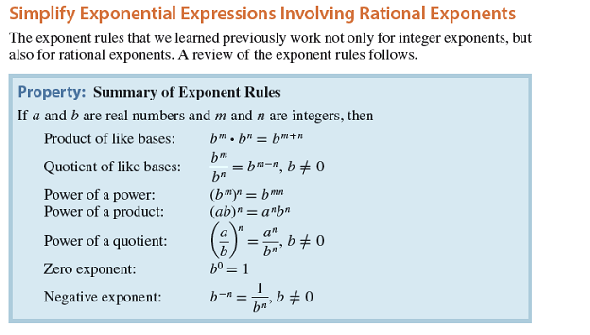
Simplify Each Expression Assume All Variables Represent P Chegg Com

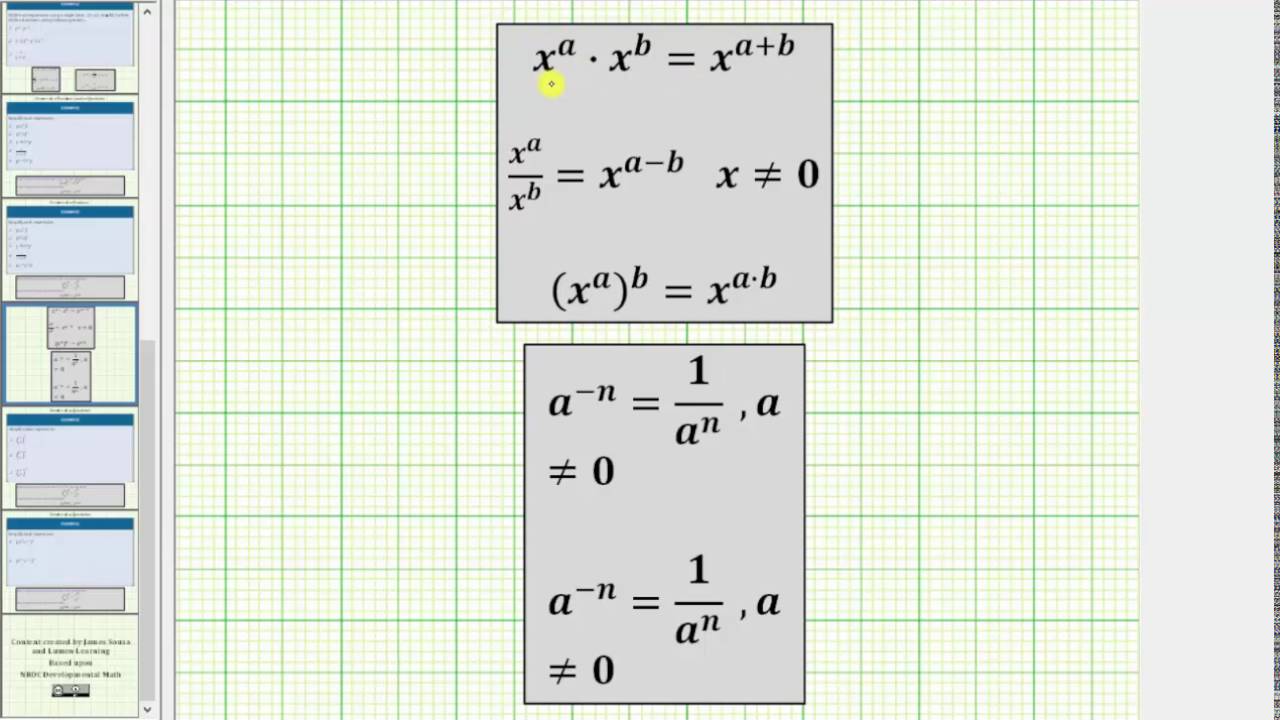
Zero And Negative Exponents College Algebra

How To Simplify Expressions With Exponents Video Lesson Transcript Study Com

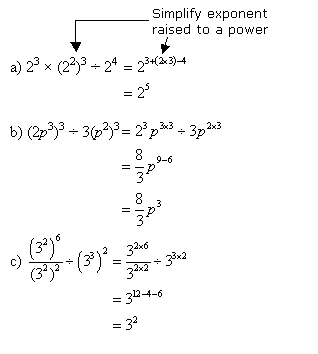
Rules Of Exponents Video Lessons Examples And Solutions

Distance Learning Simplify Expressions Using The Power Rule Exponents Unit

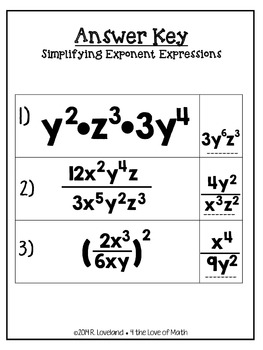
Simplifying Expressions Using Exponent Rules Powerpoint Activity